shell_一键部署PXE
本文共 1217 字,大约阅读时间需要 4 分钟。
一键部署pxe
一.shell脚本一键部署pxe
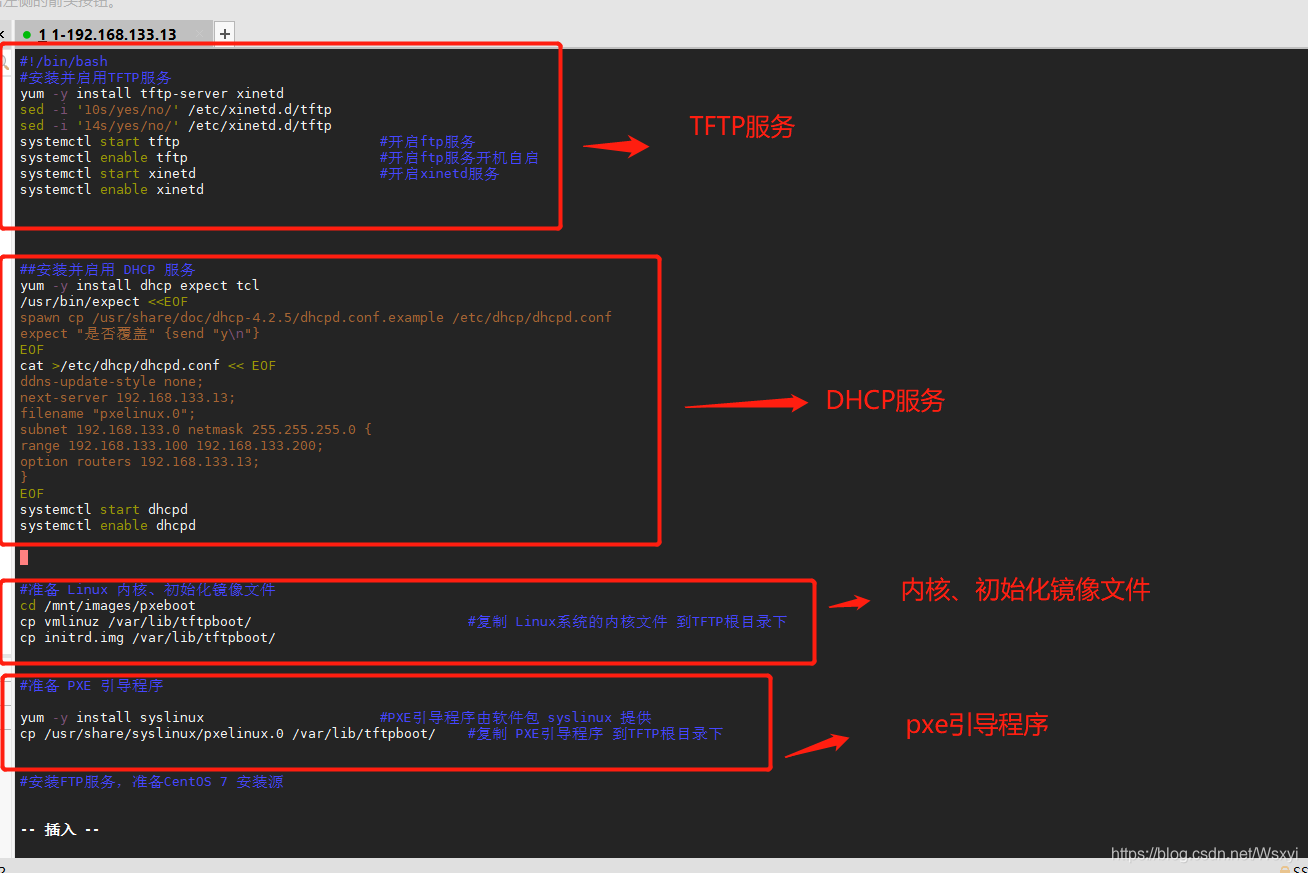
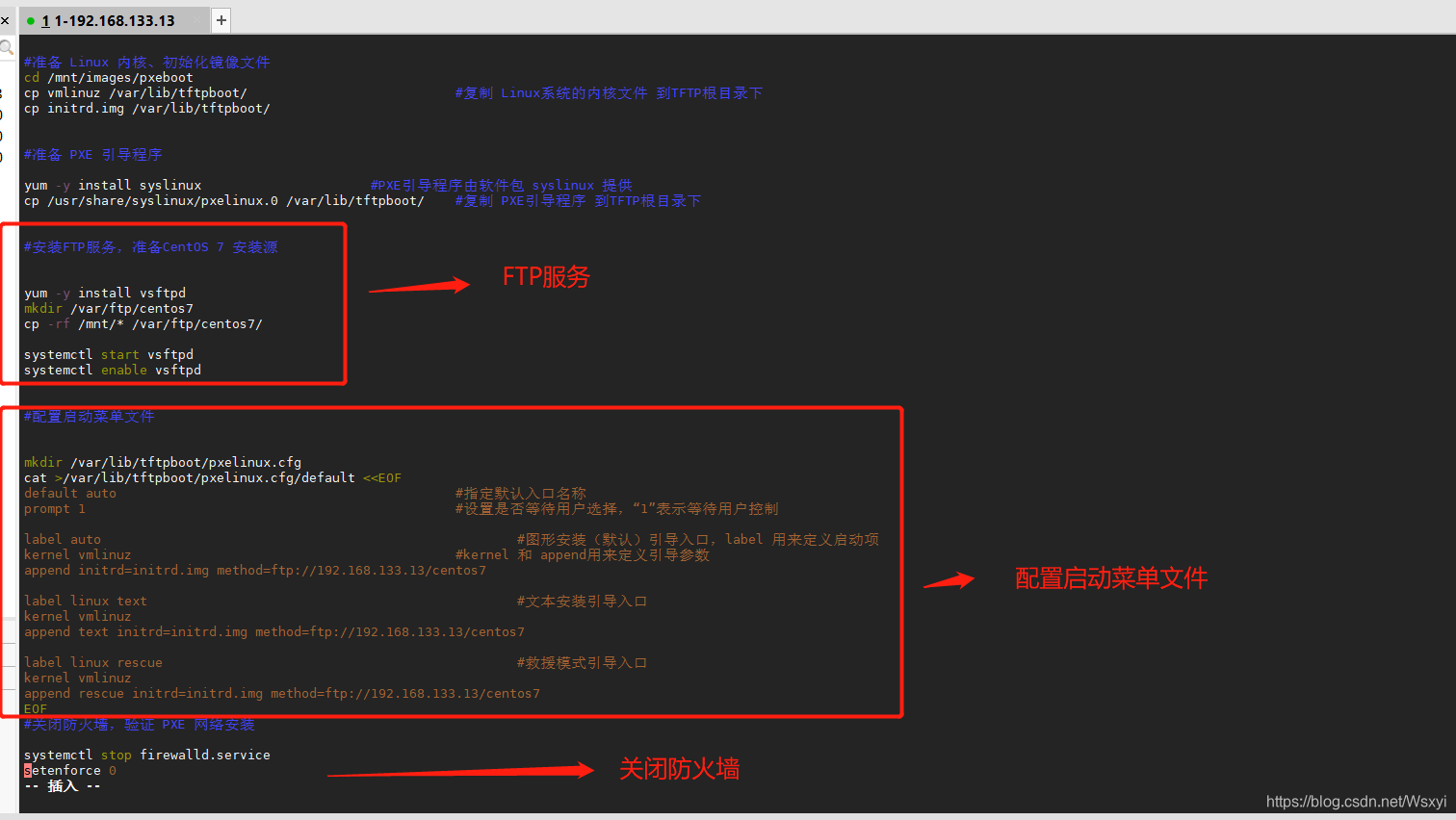
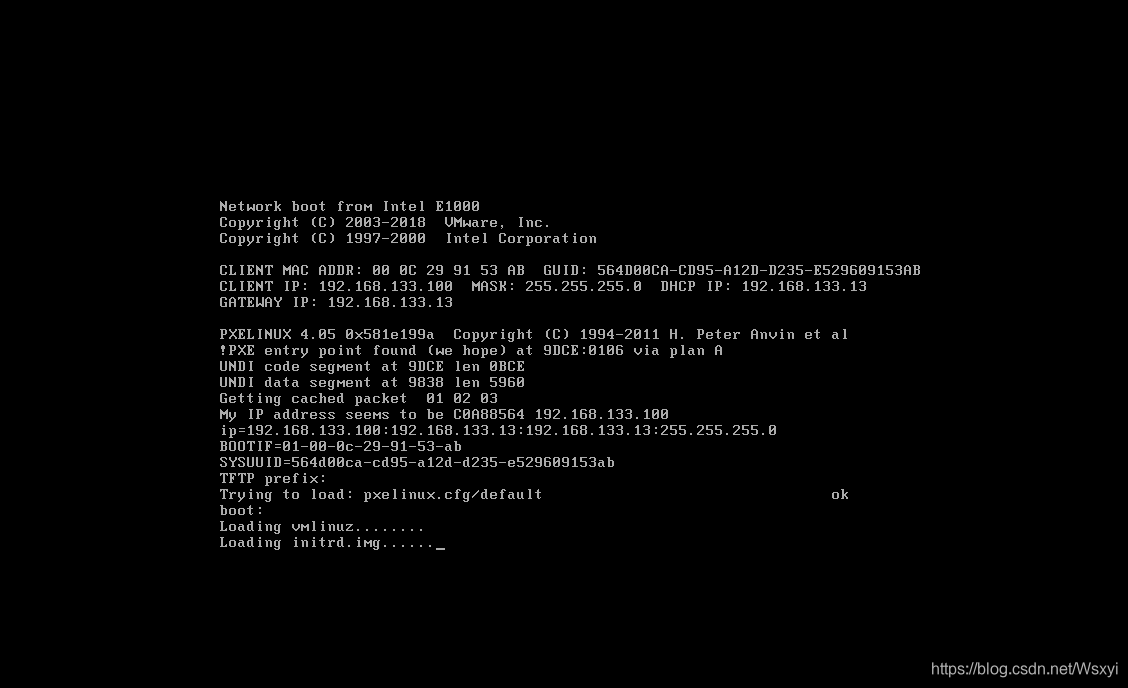
#!/bin/bash#安装并启用TFTP服务yum -y install tftp-server xinetdsed -i '10s/yes/no/' /etc/xinetd.d/tftpsed -i '14s/yes/no/' /etc/xinetd.d/tftpsystemctl start tftp #开启ftp服务systemctl enable tftp #开启ftp服务开机自启systemctl start xinetd #开启xinetd服务systemctl enable xinetd##安装并启用 DHCP 服务yum -y install dhcp expect tcl/usr/bin/expect </etc/dhcp/dhcpd.conf << EOFddns-update-style none;next-server 192.168.133.13;filename "pxelinux.0";subnet 192.168.133.0 netmask 255.255.255.0 { range 192.168.133.100 192.168.133.200;option routers 192.168.133.13;}EOFsystemctl start dhcpdsystemctl enable dhcpd#准备 Linux 内核、初始化镜像文件cd /mnt/images/pxebootcp vmlinuz /var/lib/tftpboot/ #复制 Linux系统的内核文件 到TFTP根目录下cp initrd.img /var/lib/tftpboot/#准备 PXE 引导程序yum -y install syslinux #PXE引导程序由软件包 syslinux 提供cp /usr/share/syslinux/pxelinux.0 /var/lib/tftpboot/ #复制 PXE引导程序 到TFTP根目录下#安装FTP服务,准备CentOS 7 安装源yum -y install vsftpdmkdir /var/ftp/centos7cp -rf /mnt/* /var/ftp/centos7/systemctl start vsftpdsystemctl enable vsftpd#配置启动菜单文件mkdir /var/lib/tftpboot/pxelinux.cfgcat >/var/lib/tftpboot/pxelinux.cfg/default <

转载地址:http://hseu.baihongyu.com/
你可能感兴趣的文章
MySQL5.7新增Performance Schema表
查看>>
Mysql5.7深入学习 1.MySQL 5.7 中的新增功能
查看>>
Webpack 之 basic chunk graph
查看>>
Mysql5.7版本单机版my.cnf配置文件
查看>>
mysql5.7的安装和Navicat的安装
查看>>
mysql5.7示例数据库_Linux MySQL5.7多实例数据库配置
查看>>
Mysql8 数据库安装及主从配置 | Spring Cloud 2
查看>>
mysql8 配置文件配置group 问题 sql语句group不能使用报错解决 mysql8.X版本的my.cnf配置文件 my.cnf文件 能够使用的my.cnf配置文件
查看>>
MySQL8.0.29启动报错Different lower_case_table_names settings for server (‘0‘) and data dictionary (‘1‘)
查看>>
MYSQL8.0以上忘记root密码
查看>>
Mysql8.0以上重置初始密码的方法
查看>>
mysql8.0新特性-自增变量的持久化
查看>>
Mysql8.0注意url变更写法
查看>>
Mysql8.0的特性
查看>>
MySQL8修改密码报错ERROR 1819 (HY000): Your password does not satisfy the current policy requirements
查看>>
MySQL8修改密码的方法
查看>>
Mysql8在Centos上安装后忘记root密码如何重新设置
查看>>
Mysql8在Windows上离线安装时忘记root密码
查看>>
MySQL8找不到my.ini配置文件以及报sql_mode=only_full_group_by解决方案
查看>>
mysql8的安装与卸载
查看>>